
In the next paragraph we will study some cases in more detail.This section needs additional citations for verification. Values of S and correspondingly low values of the Burgers vectors. The structure of grain boundaries, you must accept the concept of grain boundary dislocations even at rather large Why - we do not really know, although Bollmann theory does Run parallel to the lines indicated by H and J. Vectors b = a/82 and an average distance of 20 nm is visible. The following TEM picture shows a S 41 grain boundary (from Dingley andĪ network of grain boundary dislocations with Burgers Orientation still special and bound to have grain boundary dislocations? So does this still make sense? Are boundaries close to a S41 " low S" value and Burgers vectors of a/82 appear toīe a bit odd, too. Now a question might come up: S = 41 is not exactly a This is but natural - a S = 3 twin boundary is after all a very close relative to stacking faults. Lattice vectors belonging to the S = 3 boundary are our old acquaintances, the partial Burgers vectors associated with stacking Interestingly (and very satisfyingly), the DSC S i b i(DSC) = b(Lattice), we always have
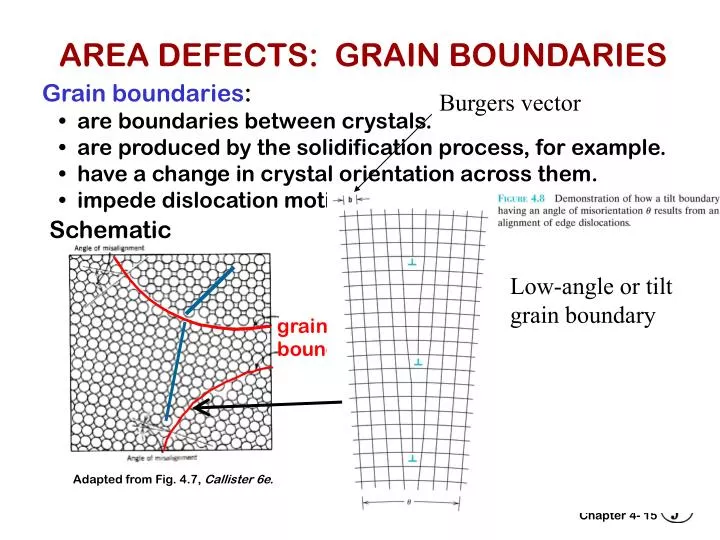
Lattice dislocations thus is always much smaller. Lattice, the energy of several DSC lattice dislocations with a Burgers vector sum equal to that of a crystal Burgers vectors of the DSC lattice are smaller than Burgers vectors of the crystal.Why should that beĭSC lattice belong to both crystals since the DSC lattice is defined Lattice that the grain boundary S endeavors to assume. Vectors of the grain boundary dislocations could be translation vectors of one of the crystals, but that isĮnergetically not favorable because the Burgers vectors are large and the energy of a dislocation scales with Gb 2 andĭislocations accounting for small deviations from a low S orientation are dislocations in the DSC lattice belonging to the CSL General preferable to dislocation-free boundaries. Which account for small misfits relative to a preferred (low) S orientation, are in Grain boundaries containing grain boundary dislocations Here we note the important pointsīetween the dislocation lines we now have a perfect S1 relation (apart from some elasticĪll of the misfit relative to a perfect S orientation is concentrated in the grain boundary dislocations.īoundary energy in the area between the dislocations and raised it along theĭislocations - there is the possibility of optimizing the grain boundary energy. We will be looking at those structures in great detail in the next paragraph. Screw dislocations in the twist case will do the necessary transformation this is schematically shown below Introducing a sequence of edge dislocations in the tilt case and a network (not necessarily square) of Grain-boundary dislocations are simple lattice dislocations. The DSC-lattice as well the CSL are identical with the crystal lattice in this case, so the Introducing grain boundary dislocations now will establish the exact S1 relation between the dislocations (and something heavily disturbed at the dislocationĬores). Obviously, we are somewhat off the S 1 position. Imagine the two extreme cases: A pure tilt and a pure twist boundary they are shown below. This kind of boundary is known as " small-angle grain boundary" ( SAGB), or, as already used above as " S1 boundary". The boundary between two crystals with almost identical orientation. Thus obtained is what you will see in a TEM picture - the crystal has no problem whatsoever to "solve"Ĭoncept, it is easiest, to consider the environment of the S= 1 grain boundary, i.e. Not to the crystal), especially when the steps possibly associated with the grain boundary dislocations must be The determination of the precise dislocation structure needed to transform aĬoincidence boundary with some superimposed grain boundary dislocation network can be exceedingly difficult (to you,

7.2.1 Small Angle Grain Boundaries and Beyond 7.2 Grain Boundaryĭislocations 7.2.1 Small Angle Grain Boundaries and Beyond


 0 kommentar(er)
0 kommentar(er)
